The Solar Dynamo: The Physical Basis of the Solar Cycle and the Sun’s Magnetic Field
In my previous article, I laid out some basics about the sun’s structure and physical characteristics in order to set up the groundwork upon which I could then explain the physical mechanism which underlies the solar cycles I talked about in the article prior to that one. I understand that this is a bit more technical than most readers may be accustomed to, which is why I’ve included a simplified “tl; dr” version before delving deeper.
Solar Dynamo Theory
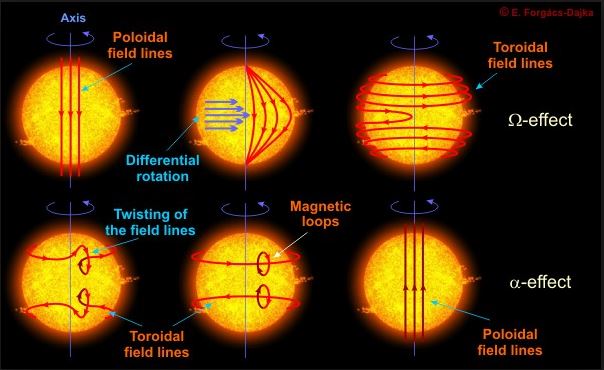
The leading scientific explanation for the mechanism by which these solar cycles emerge is the solar dynamo theory. It arises from an area of physics called magnetohydrodynamics, which is the field which studies the magnetic properties of electrically conducting fluids, and is covered in most university textbooks on plasma physics. So how does it work?
The tl; dr version is as follows: (more…)